One of the most important topics in elementary calculus is the  definition of limits. The definition says that the
definition of limits. The definition says that the  if and only if, for all
if and only if, for all 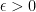 , there exists a
, there exists a  such that if
such that if  , then
, then  . In this article, we are going to discuss what this definition means. Readers of this article must have knowledge about elementary calculus and the concept of limits.
. In this article, we are going to discuss what this definition means. Readers of this article must have knowledge about elementary calculus and the concept of limits.
Review of Limit Basics
Consider the function 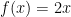 . We have learned from elementary calculus that
. We have learned from elementary calculus that 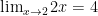 . Aside from algebraic computation, this is evident from the color-coded graph and the table shown in Figure 1. The yellow arrows in the graph and the values in the yellow cells in the table indicate that as the value of
. Aside from algebraic computation, this is evident from the color-coded graph and the table shown in Figure 1. The yellow arrows in the graph and the values in the yellow cells in the table indicate that as the value of  approaches
approaches 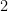 from the left of the x-axis, the value of
from the left of the x-axis, the value of  approaches
approaches  from below of the y-axis. On the other hand, the red arrows in the graph and the values in the red cells in the table indicate that as the value of
from below of the y-axis. On the other hand, the red arrows in the graph and the values in the red cells in the table indicate that as the value of  approaches
approaches 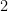 on from the right of the x-axis, the value of
on from the right of the x-axis, the value of  approaches
approaches  from above of the y-axis.
from above of the y-axis.

Figure 1 – The table and the graph showing the value of f(x) as x approaches 2 from both sides.
From the above discussion, it is noteworthy to mention three things:
- We can get
 as close to
as close to  as we please by choosing an
as we please by choosing an  sufficiently close to
sufficiently close to 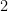 . For example, I can set
. For example, I can set  to
to  (with
(with 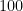 nines) to get an
nines) to get an  very close to
very close to  , which is
, which is 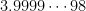 (
(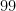 nines).
nines).
- No matter how small is the distance of
 from
from 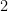 , a distance less than it may still be chosen. For example, if we choose the point which is very close to
, a distance less than it may still be chosen. For example, if we choose the point which is very close to 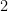 , say a point with coordinate
, say a point with coordinate 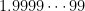 with (
with (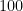 nines), we can still choose a value closer than this to
nines), we can still choose a value closer than this to 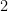 . For instance, we can choose
. For instance, we can choose 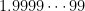 with
with 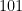 nines. This can be repeated for every chosen distance.
nines. This can be repeated for every chosen distance.
- Although
 can be very very close to
can be very very close to 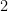 , it does not necessarily mean that
, it does not necessarily mean that  equals
equals 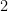 .
.
Now we go back to the definition of limits. In a specific example, the limit definition states that the 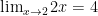 if (and only if) for all distance (denoted by the Greek letter
if (and only if) for all distance (denoted by the Greek letter  ) from
) from  along the y-axis (directly above or below
along the y-axis (directly above or below  ) – no matter how small – we can always find a certain distance (denoted by
) – no matter how small – we can always find a certain distance (denoted by 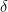 ) from
) from 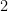 along the x-axis (left or right of
along the x-axis (left or right of 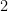 ) such that if
) such that if  is between
is between  and
and 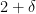 , then
, then  would lie between
would lie between  and
and  .
.
To give you a more concrete example, suppose we want the distance  from
from  , which is our limit, to be
, which is our limit, to be  then the interval of our
then the interval of our  is (
is (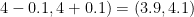 . The definition of limit says that given a distance
. The definition of limit says that given a distance 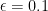 , we can find a distance
, we can find a distance 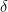 in the x-axis such that if
in the x-axis such that if  is between
is between  and
and 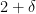 , we are sure that
, we are sure that  is between
is between  and
and  . We do not know the value of
. We do not know the value of 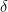 yet, but we will calculate it later.
yet, but we will calculate it later.
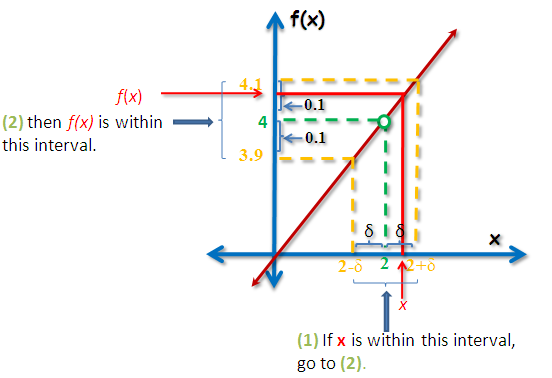
Figure 2 – The epsilon-delta definition given epsilon = 0.1.
In Figure 2,  is between
is between  and
and 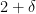 or
or  . Subtracting
. Subtracting 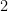 from all terms of the inequality, we have
from all terms of the inequality, we have 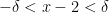 . If you recall the definition of absolute value, this is precisely the same as
. If you recall the definition of absolute value, this is precisely the same as 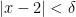 . The comparison among the notations is in Table 1.
. The comparison among the notations is in Table 1.
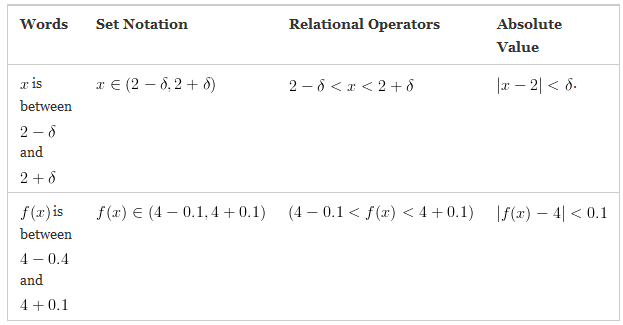
Using the notations in the table, we can conclude that the following statements are equivalent:
- Words: Given
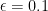 , we can find a
, we can find a 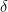 such that if
such that if  is between
is between 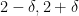 , then
, then  is between
is between  and
and  .
.
- Set Notation: Given
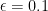 , we can find a
, we can find a 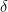 such that if
such that if  , then
, then 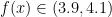 .
.
- Relational Operator: Given
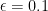 , we can find a
, we can find a 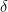 such that if
such that if  , then
, then  .
.
- Absolute Value: Given
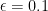 , we can find a
, we can find a 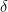 such that if
such that if  , then
, then  .
.
We have discussed that we can get  as close to
as close to  as we please
as we please
by choosing an  sufficiently close to
sufficiently close to 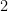 . This is equivalent to choosing an extremely small
. This is equivalent to choosing an extremely small  , no matter how small, as long as
, no matter how small, as long as  . Our next task is to find the
. Our next task is to find the 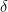 that corresponds to that
that corresponds to that  .
.
Applying this definition to our example, we can say the 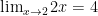 if and only if, given
if and only if, given 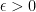 (any small distance above and below 4), we can find a
(any small distance above and below 4), we can find a 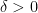 (any distance from x to the left and right of
(any distance from x to the left and right of 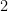 ) such that if
) such that if 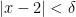 , then
, then  .
.
The Definition of a Limit of a Function
Now, notice that  is the limit of the function as
is the limit of the function as  approaches
approaches 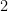 . If we let the limit of a function be equal to
. If we let the limit of a function be equal to 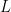 and
and  be the fixed value that
be the fixed value that  approaches, then we can say that
approaches, then we can say that  if and only if, for any
if and only if, for any  (any small distance above and below
(any small distance above and below 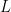 ), we can find a
), we can find a  (any small distance from to the left and to the right of a) such that if
(any small distance from to the left and to the right of a) such that if  then,
then, 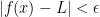 . And that is precisely, the definition of limits that we have stated in the first paragraph of this article.
. And that is precisely, the definition of limits that we have stated in the first paragraph of this article.
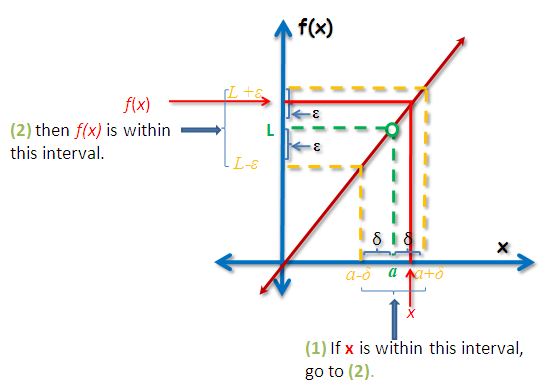
Figure 3 – The epsilon-delta definition given any epsilon.
In mathematics, the phrase “for any” is the same as “for all” and is denoted by the symbol  . In addition, the phrase “we can find” is also the same as “there exists” and is denoted by the symbol
. In addition, the phrase “we can find” is also the same as “there exists” and is denoted by the symbol  . So, rephrasing the definition above, we have
. So, rephrasing the definition above, we have  if and only if,
if and only if,  , such that if
, such that if  then,
then, 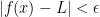 . A much shorter version of this definition is the phrase
. A much shorter version of this definition is the phrase  , such that
, such that  . The symbol
. The symbol  stands for if and only if and the symbol
stands for if and only if and the symbol  is similiar to if-then. If
is similiar to if-then. If 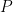 and
and 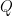 are statements, the statement
are statements, the statement 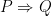 is the same as the statement of the form “If
is the same as the statement of the form “If 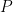 then
then 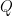 “.
“.
Finding a specific delta
We said that given any positive  , we can find a specific
, we can find a specific 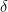 , no matter how small our
, no matter how small our  is. So let us try our first specific value
is. So let us try our first specific value 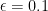 .
.
From the definition, we have 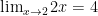 if and only if, given
if and only if, given 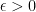 (any small distance above and below 4),
(any small distance above and below 4),  such that if
such that if  then,
then,  .
.
Now 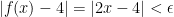 . This implies that
. This implies that
 which implies that
which implies that  . Simplifying, we have
. Simplifying, we have  . This means that our
. This means that our  should be between
should be between  and
and 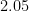 to be sure that our
to be sure that our  is between
is between  and
and  . This is shown in Figure 4.
. This is shown in Figure 4.
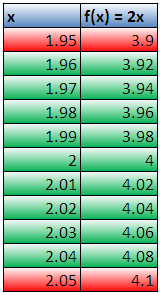
Figure 4 – The table showing some of the values of epsilon and delta satisfying the definition of limit of 2x as x approaches 2.
Now, let  . This means that our interval is
. This means that our interval is 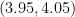 . Now
. Now 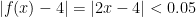 . Thus,
. Thus, 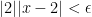 which implies that
which implies that 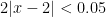 . Solving, we have
. Solving, we have 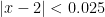 . This means that our
. This means that our  should be between
should be between 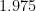 and
and  to be sure that our
to be sure that our  is between
is between  and
and  . There are only two examples above, but the definition tells us that we can choose any
. There are only two examples above, but the definition tells us that we can choose any 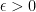 so let us generalize our statement by doing so.
so let us generalize our statement by doing so.
Now 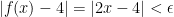 . This results to
. This results to 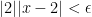 which implies that
which implies that  . Solving, we have
. Solving, we have 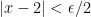 . From the condition above,
. From the condition above, 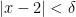 so we can let
so we can let 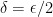 .
.
This means given any  , we just let our
, we just let our 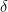 equal to
equal to  and we are sure that if
and we are sure that if  is between
is between  and
and  to be sure that our
to be sure that our  is between
is between  and
and  .
.
In the next calculus post, we are going to discuss the strategies on how to get 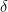 given an arbitrary
given an arbitrary  value, so keep posted.
value, so keep posted.
Related Articles