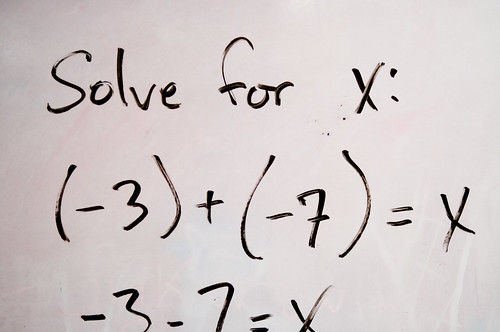Guest Post: Why Study Algebra

High School Algebra, High School Mathematics, Math in Real Life

Many students who begin learning Algebra and even the ones who have spent many years learning Algebra have a common question: Why study this? Where will this be used? Why are there letters like x, y, z used instead of numbers in Math?  In this article we will try to address this question.

algebra

We all directly or indirectly use Algebra in our everyday life and most of the times unknowingly. For those who have spent some years with algebra will find k \times xy too algebraic but immediately recognize  \frac{1}{2} \times b \times h as the formula for the area of a triangle. Though one can see that conceptually both the expressions are identical (with one constant and two variables) and algebraic, one instantly connects with the usefulness of an expression or a formula than its abstract counterpart.

Algebra has many direct applications. In this article let us just discuss a few financial/money applications.

Everyone uses money. Many pay installments on their cars or home, many invest money in fixed deposits or recurring deposits.

One can calculate the future value (FV) of an investment/fixed deposit (PV) (present value)) at a given rate of interest i for a given period of time (n) by using the formula:

 FV = PV(1+i)^n

The above formula uses exponentials. When we started learning them we thought that it was just for fun! Remember here i is a fraction. For example 2% is written as 0.02.

But if one wants to know the rate of interest the bank gives then the formula would look like

i = \left(\displaystyle\frac{FV}{PV} \right)^{\frac{1}{n}} - 1

or, if one wishes to know the number of years/months for which he needs to invest the money to get an expected future value, using simple algebra they can determine that the number of years

n = \displaystyle\frac{\log \left(\frac{FV}{PV} \right)}{\log(1 + i)},

The above formula uses logarithms; again once studied in High School.

Rule of 72:

Now, if one wants to know the number of years that will be required to double the money (\frac{FV}{PV} = 2) then n = \frac{\log 2}{log(1 + i)}. This function can be approximated using the Rule of 72,

n = \displaystyle\frac{0.72}{r}.

For example if i = 0.09 per annum, then the number of years required to double the money is \frac{0.72}{0.09} = 8 years. In fact a more accurate answer would be \frac{\log(2)}{\log(1+1.09} \approx 8.0432 years. Now this answer is very close to the approximate answer and thus, Rule of 72 can be used for quick calculations.

Future Value of an Investment

Suppose you open a Recurring Deposit Account in a bank and deposit an amount A every month for n number of months (number of years multiplied by 12) and the bank pays you a monthly interest of i (annual interest divided by 12). The amount that you deposit gets compounded every month. That means that you get interest on the deposits and on the previous deposits and the accrued interest and thus the calculation becomes complex. Here, Algebra comes to our rescue and we can derive a formula to find the same. Now the derivation, not discussed here, uses concepts of Algebra like the Geometric Series and other simple Algebraic concepts.

The formula of Future Value of the periodic investment A deposited at the end of the month is

FV = \frac{A}{i}((1+i)^n - 1)

And if you want to find the Future Value of the periodic investment A deposited at the start of the month, just multiply  to the above formula since an interest of an extra month is added.

EMI

EMI stands for Equated Monthly Installments. It is the periodic installment A paid at the end of the month to the bank/lender on a Loan Amount PV. Now we have studied two formulae:

FV = PV(1+i)^n

FV = \frac{A}{i}((1 + i)^n - 1)

Equating both the equations we get

PV(1+i)^n = \frac{A}{i}(1 + i)^n - 1

And isolating A (which will give us the EMI) we get

A = \displaystyle\frac{PV \times i \times (1 + i)^n}{(1 + i)^n - 1}

Also we can isolate PV to calculate the Present Value of the investment

PV = A\left(\displaystyle\frac{(1+i)^n-1}{1 \times (1+i)^n} \right)

Now again some (those who are lazy/disinterested in learning Algebra) may argue that the above mentioned formulae were important when the computers were not invented. But nowadays, there are various softwares like MS Excel that does all the calculations and we need not know Algebra or know any formula to find things like EMI. The answer to them is that the computer software use either the direct formula that has been derived using Algebra or use Algorithms and programming, which again makes use of Algebra and logic. A programmer makes use of variables which again is an Algebraic concept.

So, in other words if our ancestors hadn’t invented Algebra, the higher level concepts would have had never developed. There would be no computers, no mobile phones or anything that makes use of higher Mathematics and Sciences. Even Statistics makes use of Algebra and Statistics are used by all most everyone including businesspeople, researchers, etc. If you do not know this, you are yet to study them.

You in fact are more fortunate than the past generations. You have internet to discover more usefulness of Algebra, Geometry, Calculus and other subjects. So be assured that everything you learn at school is useful and will be helpful, directly or indirectly. So take interest in studying and you will enjoy the studies.

The Author

My name is Sohael Babwani from Mumbai, India. I give private tuitions to schoolchildren. I teach all subjects including Maths. In the year 2004, I had written an article “An Extended Approach to the Julian and the Gregorian Calendar.” This article was published in The Mathematical Gazette, London. I have made a blog/website http://www.babwani-congruence.blogspot.in/ explaining the same.

***

Photo Credit: jronaldlee via Flickr

2 comments , , , , , ,

Leave a Reply