Why Finger Multiplication Works
In the Finger Multiplication post, we have learned how we can cleverly multiply numbers from 6 to 10 using our fingers without memorizing the multiplication table. In this post, we will discuss the reason why this method works. The proof can be written in a few lines, but since this article falls under the elementary school mathematics category, we will discuss it with more details.
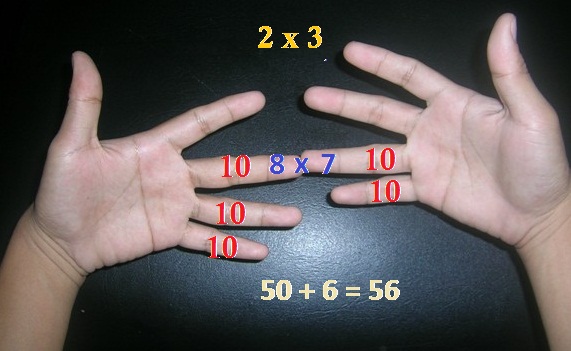
Finger Multiplication of 8 x 7.
Let’s examine the first example in the previous article as shown above. In the example, we wanted to multiply 8 by 7, so we connected the ring and the middle fingers. In this discussion, we will call the connected fingers and all the fingers below them down fingers, while we call all the fingers above them up fingers.
The Down Fingers
There are 3 down fingers on the left hand and 2 down fingers on the right (see figure). We multiplied each of them by 10, and added them up, which equaled 50. Using 8 , 7, and 5 (the total number of fingers on one hand), we can relate down fingers as shown in the following equation: 10(2) + 10(3) = 10(8-5) + 10(7-5). Can you see why?
The Up Fingers
Looking at the up fingers we have 2 at the left and 3 at the right and we multiplied them to get 6. Note that we can also relate them to 10 (the total number of fingers) as well as 8 and 7, as shown in the following equation: 2 x 3 = (10-8) x (10 – 7).
Adding them up and Generalizing
Using the equations above, we can express the product P of 8 and 7 using the following equation:
P = [10(8-5) + 10(7-5)] + [(10-8) x (10 – 7)] = 56
As an exercise, verify if this method works in the second example in the previous article.
Generalizing, what if we want to multiply the numbers m and n?
We now generalize by multiplying m and n. The product P is given by the equation P = [10(m-5)+10(n-5) + (10-m)(10-n)]. Simplifying the equation we have P = 10m – 50 + 10n – 50 + (100 – 10m – 10n + mn) = mn. But mn is the product of m and n, which is what we want to show. That is the reason why the method works.