GeoGebra Tutorial 18 – Area Under a Curve and Riemann Sums
This is the 18th tutorial of the GeoGebra Intermediate Tutorial Series. If this is your first time to use GeoGebra, I strongly suggest that you read the GeoGebra Essentials Series.
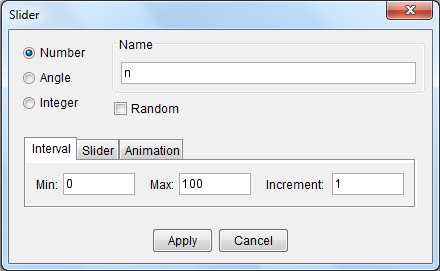
In this tutorial, we compare the area of a plane under a curve f(x) = x2 bounded by the x-axis, the y-axis, and the line x = 1 with the sum of the areas of rectangular partitions under the same boundaries. We use the Slider tool to increase the number of rectangles and observe how they relate to the actual area.
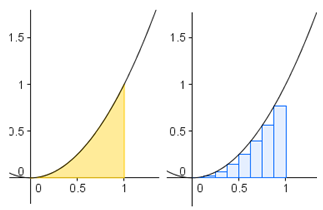
Figure 1 – Actual and approximate area bounded by the curve, the x-axis and x=1
If you want to follow this tutorial step-by-step, you can open the GeoGebra window in your browser by clicking here. You can view the output of this tutorial here.
| 1. Open GeoGebra and select Algebra and Graphics from the Perspectives menu. | |
| 2. Graph f(x) = x2 by typing f(x) = x^2 in the Input bar and press the ENTER key on your keyboard. | |
| 3. We now create a slider for the number of rectangles. Select the Slider tool and click on the Graphics view.
In the Slider dialog box, change the name to n, set the minimum to 0, maximum to 100, and increment of 1, then click the Apply button. |
|
6. To construct the lower sum (rectangles whose upper left corners are on the curve), type lowersum[f,0,1,n] and then press the ENTER key on your keyboard. That is, the lowersum (sum of the areas of rectangles) under the function f from 0 to 1 with n number of rectangles.
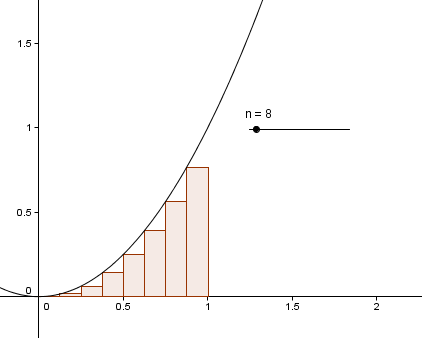 Figure 3 – Approximate area with n = 8.
|
|
| 7. Move Slider n. What do you observe? Move n to the extreme right. What is the value of the lower sum or the total are of the rectangles under the curve? | |
| 8. To construct the upper sum, type uppersum[f,0,1,n] in the drawing pad, and press the ENTER key. | |
| 9. Right click a (the value of the lowersum) in the Algebra window, and click Object Properties to show the Preferences window. | |
10. In the Preferences window, select the Color tab, choose a different color, then click the Apply button. This will make it easier to distinguish the two sums.
 Figure 4 – Approximate area of the lower sum and the upper sum. |
|
| 11. Move the slider to 100. What do you observe about the values of the the upper sum and the lower sum. Explain why your observation is such. | |
| 12. To get the actual area under the curve, we need the integral of the function f from 0 to 1. To do this, type integral integral[f, 0, 1] in the Input bar, and press the ENTER key. | |
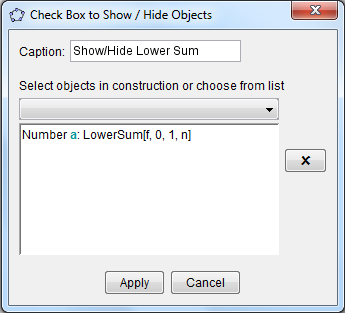
| 13. Next, we construct a check box that will show/hide the three objects. To do this, select the Check box tool and click anywhere on the Graphics view. | |
| 14. In the Caption text box, type Show/Hide Lower Sum, select Number a: Lower Sum[f,0,1,n] in the Select objects… box, and then click the Apply button. | |
| 15. Using steps 13-14 to create two more Show/Hide Check boxes for the Upper Sum and the actual area (integral of f from 0 to 1). | |
| 16. How are the lower sum, upper sum and area under the curve related? | |
| 17. How are the upper sum and lower sum related to the number of rectangles? |