GeoGebra Tutorial 9 – Vector and Translation
This is the ninth tutorial in the GeoGebra Intermediate Tutorial Series. If this is your first time to use GeoGebra, you might want to read the GeoGebra Essentials Series.
In this tutorial, we use the Vector between Two Points tool to translate a triangle and investigate the relationship between its preimage and image. We will also use the grid in this tutorial.
If you want to follow this tutorial step-by-step, you may open the GeoGebra window in your browser by clicking here.
| 1.) Open GeoGebra and select the Algebra & Graphics view from the Perspectives menu. | |
| 2.) Display the grid by clicking the View menu and choosing Grid. | |
| 3.) Click the New Point tool and place the points on the coordinates given: A on (2,3), B on (4,1) and C on (5,2). | |
| 4.) Next, we draw triangle ABC using the Polygon tool. To do this, click the Polygon tool and click the points in the following order: point A, point B, point C and point A again to close the polygon. | |
| 5.) To display the label and the coordinates of the points, right click the points then click Object Properties to display the Preferences dialog box. | |
6.) In the Basic tab of the Preferences dialog box, check the Show label check box, and choose the Name & Value option in the drop-down list box, and then close the window. Your drawing should look like the figure below.
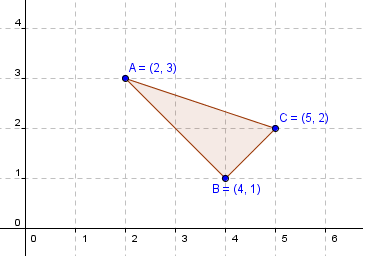 Figure 1 |
|
7.) The only remaining part of the construction is the vector tool. To construct vector DE, select the Vector between Two Points tool, click the origin and click the coordinate (1,2). After this step, your drawing should look like the one shown in Figure 2.
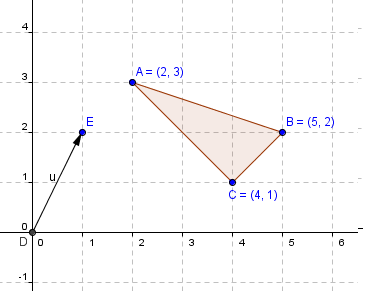 Figure 2 |
|
| 8.) To translate the object using the vector, select the Translate Object by Vector tool, click the triangle and then click the vector. Notice that a translated triangle appears after clicking the vector tool. | |
| 9.) What can you say about the preimage of the triangle object and the translated object? | |
| 10.) If the coordinates of the vertices of the translated triangle is not displayed, display it using the steps we have done in step 5 and 6. | |
| 11.) What do you observe about the relationship of the coordinates of the points of the original triangle and the translated triangle? | |
| 12. Move the terminal point (point E) of the vector. Does your observation in (11) still hold? |