Geometer’s Sketchpad Essentials 4 – The SSS Triangle Congruence
This is the fourth part of the Geometer’s Sketchpad Essentials Series. In this tutorial, we are going to construct another triangle which is congruent to a given triangle using the concept of the SSS triangle congruence. Recall that the SSS congruence theorem tells us that two triangles are congruent, if their corresponding sides are congruent. In doing the construction, we are going to learn how to use the Ray tool, the Circle tool, and other commands.
1.) Construct triangle ABC.
2.) Next, we construct ray DE. To do this, click the Straightedge tool box and hold the mouse button to display the other tools. Now, choose the Ray tool.
3.) Click two distinct points on the sketch pad and display the names of the two points. Your sketch should look like the first figure.
 4.) Next, we will construct a segment DF which is congruent to AC. To do this, be sure to deselect all the objects by clicking on the vacant part of the sketch pad. Select point D, then select segment AC (do not select the points!), click the Construct menu, and then click Circle By Center+Radius. This will produce a circle with center D and radius equal to the length of AC.
4.) Next, we will construct a segment DF which is congruent to AC. To do this, be sure to deselect all the objects by clicking on the vacant part of the sketch pad. Select point D, then select segment AC (do not select the points!), click the Construct menu, and then click Circle By Center+Radius. This will produce a circle with center D and radius equal to the length of AC.
5.) Intersect the circle and the ray by selecting both objects, clicking the Construct menu and the selecting Intersection. Move point C or A and observe how the radius of the circle adjusts.
6.) Next we hide the circle. To do this, be sure that the circle (and no other object) is selected, click the Display menu from the menu bar, and then click Hide Circle.
7.) We now copy AB. This means that we will create a circle with center D and radius equal to the length of AB. To do this, select point D, select AB, click Construct, and then click Center By Center+Radius.
8.) Next, we copy the third side BC. We construct the circle with center F and radius BC. To do this, select point F and segment BC, and click Construct, and then click Center By Center+Radius. Your sketch should look like the second figure. Note that one of the intersections of the circles is our third vertex. 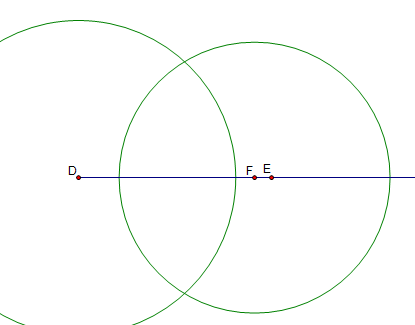 9. Using the Construct>Intersection command will give us two intersections, but we only need one. To do this, click the Point tool, hover over the intersection, and click when the two circles are highlighted. Name the intersection G.
9. Using the Construct>Intersection command will give us two intersections, but we only need one. To do this, click the Point tool, hover over the intersection, and click when the two circles are highlighted. Name the intersection G.
10.) To complete the triangle, construct segments DG and FG, and hide the two circles. Hide the ray, point E, and connect DF to complete the activity. Your sketch should have two congruent triangles.
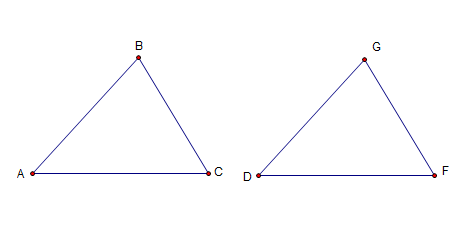 11. Move the points on both triangles. What do you observe? Explain why your observations are such.
11. Move the points on both triangles. What do you observe? Explain why your observations are such.