Derivation of the Quadratic Formula
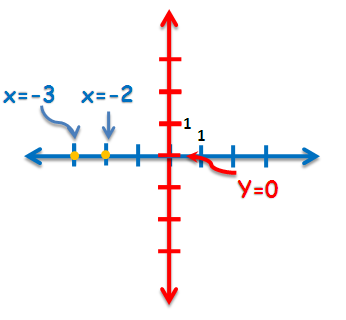
When we discuss about functions, we usually talk about their roots, or geometrically where their graphs pass through the x-axis. For example, and
are the roots of the graph of the function in Figure 1 because it passes through
and
.
Since we are looking for points on the x-axis, it means that all the points that we are looking for have -coordinate
. As a consequence, (i) if we want to find the root of a quadratic function we have set
and then solve for the values of
.
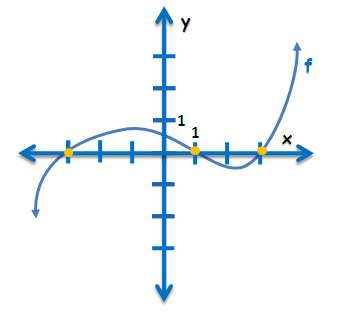
Figure 1 – The x-axis and the line with equation y = 0 are basically the same line so all points on the x-axis have y-coordinate 0.
With the things above in mind, let us find the roots of two quadratic functions: and
.
Finding the root by Factoring
The roots of the function are easy to find. As we have said, to get the root of a function, we set
to
and then find the value the value of
. Solving by factoring, we have
Now, if
or/and
. Solving for the value of
on both equations, we have
and
.
Thus, even though we have not seen the graph of the function yet , we are sure that it will pass through
and
. If you want to verify if the graph of the function
indeed passes through the
-axis at
and
, you can verify its graph using a graphing software or a graphing calculator.
Factoring: Another Interpretation
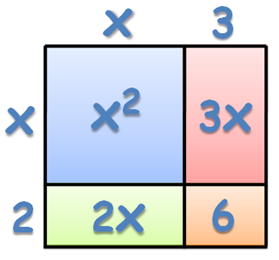
Another possible interpretation of the expression can be the area of a rectangle with length
and width
. The distribution of the products of the terms of the expressions are represented by the four rectangles formed shown below.
Let us try another example: Let us find the roots of the quadratic function . You will probably observe that there is no way that we can factor this expression. The last term is
, but there are only two factors of 1:
and
, so this means that that the numerical coefficient of
must be
or
, but it is equal to
. Hence, the expression is not factorable.
Since, the expression is not factorable, we cannot find the length and width of a rectangle with area . The easiest way probably to find its length and width is to assume that it is a square.
Completing the Square
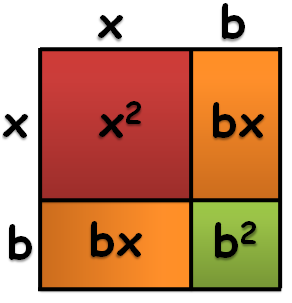
We have a quadratic expression which we assumed a perfect square so its factor must be of the form where
is a real number. Also,
. If we consider
as a side of the square, then the product of the expressions will form two squares namely
and
, and 2 congruent rectangles with each having an area of
.
If we want to use the product of above, first, we have to take off
as one of the squares. Then we are left with a figure with area
which we will divide into two congruent rectangles. If we are going to follow the positions of the rectangles in Figure 4, then we will have an
and two pieces of
(see Figure 5).To construct a square, we extend one of the sides of each of the congruent rectangles.
Since we have two small rectangles with area , and the longer side (in the diagram) is
, it follows that the other dimension is
which gives us a smaller square with area
.
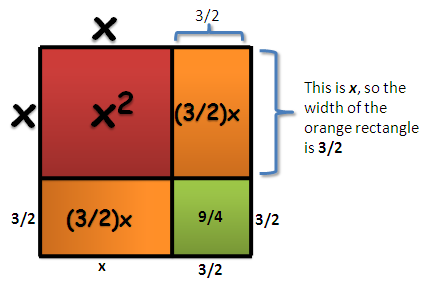
Figure 6 – The area of the small formed is 9/4 and the side length of the big square formed is x + 3/2.
The biggest square formed in Figure 6 has area , which is
more than our original quadratic expression, so we will deduct
to preserve the original expression. So our final expression is
Algebraically, if we have the expression , and we want to “compete its square”, we want to transform it to an expression of the form
. For example,
can be expressed as
. Another example is that
can be written as
. Note that the coefficient of
should be
so that we are sure that a square
by
is formed as shown in figures 4,5 and 6. In general, the possible steps that we are going to create using the general equation
is to set
to
and then find the value of
.
In constructing the square in Figure 6, we went through the following processes:
(ii) We made sure that the numerical coefficient of is
to ensure that we have a square with factors (side length)
.
(iii) We isolated the constant term , and we just used the first
and the second term
.
(iv) To get the area of the smaller square, we divided the numerical coefficient of the by
then squared it to get
.
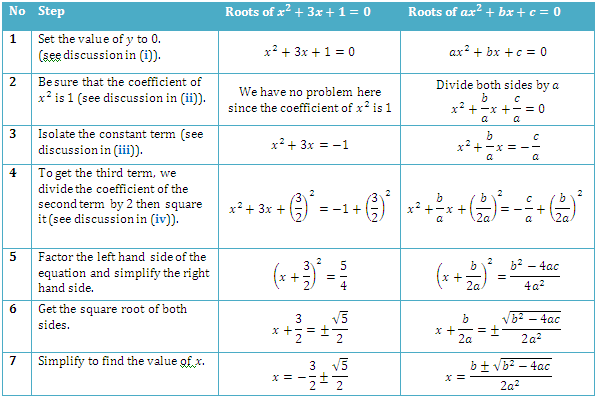
Shown in Figure 7 is the summary of the steps we did to get the roots of the quadratic function . The rightmost column of the table shows the generalization of our steps, which is getting the roots of the quadratic function
.
Quadratic Formula
The formula located at the bottom part of the rightmost column of the table in Figure 7 is called the quadratic formula. We have derived the quadratic formula from completing the square of a quadratic equation. From the formula, the roots o the quadratic function
are
and
.