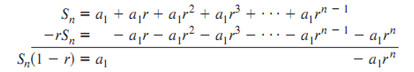Introduction
We have discussed about arithmetic sequences, its characteristics and its connection to linear functions. In this post, we will discuss another type of sequence.
The sequence of numbers 2, 6, 18, 54, 162, … is an example of an geometric sequence. The first term 2 is multiplied by 3 to get the second term, the second term is multiplied by 3 to get the third term, the third term is multiplied by 3 to get the fourth term, and so on. The same number that we multiplied to each term is called the common ratio. Expressing the sequence above in terms of the first term and the common ratio, we have 2, 2(3), 2(32), 2(33), …. Hence, a geometric sequence, also known as a geometric progression, is a sequence of numbers where each term after the first is found by multiplying the previous one by a fixed non-zero number called the common ratio.
The Sierpinski triangle below is an example of a geometric representation of a geometric sequence. The number of blue triangles, the number of white triangles, their areas, and their side lengths form different geometric sequences. It is left to the reader, as an exercise, to find the rules of these geometric sequences.
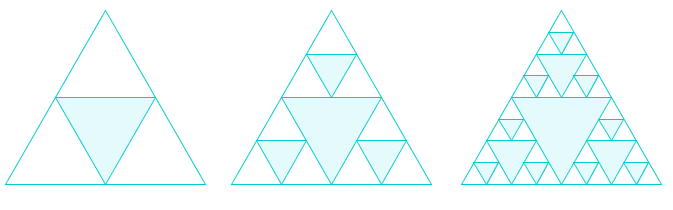
Figure 1 - The Seriepinski Triangles.
To generalize, if a1 is its first term and the common ratio is r, then the general form of a geometric sequence is a1, a1r, a1r2, a1r3,…, and the nth term of the sequence is a1rn-1.
A geometric series, on the other hand, is the sum of the terms of a geometric sequence. Given a geometric sequence with terms a1r, a1r2, a1r3,…, the sum Sn of the geometric sequence with n terms is the geometric series a1 + a1r + a1r2, a1r3 + … + arn-1. Multiplying Sn by -r and adding it to Sn, we have
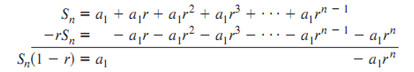
Hence, the sum of a geometric series with n terms, and 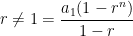 .
.
Sum of Infinite Geometric Series and a Little Bit of Calculus
Note: This portion is for those who have already taken elementary calculus.
The infinite geometric series 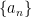 is the the symbol
is the the symbol  . From above, the sum of a finite geometric series with
. From above, the sum of a finite geometric series with 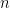 terms is
terms is  . Hence, to get the sum of the infinite geometric series, we need to get the sum of
. Hence, to get the sum of the infinite geometric series, we need to get the sum of  . However,
. However, 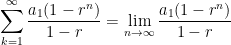 .
.
Also, that if  ,
,  approaches
approaches  (try
(try  or any other proper fraction and increase the value of
or any other proper fraction and increase the value of 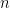 ), thus,
), thus, 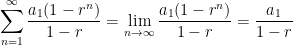 . Therefore, sum of the infinite series
. Therefore, sum of the infinite series 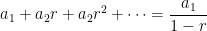 .
.
One very common infinite series is 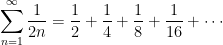 , or the sum of the areas of the partitions of the square with side length 1 unit shown below. Using the formula above,
, or the sum of the areas of the partitions of the square with side length 1 unit shown below. Using the formula above,
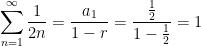 .
.

Figure 2 - A representation of an infinite geometric series.
This is evident in the diagram because the sum of all the partitions is equal to the area of a square. We say that the series 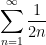 converges to 1.
converges to 1.