half angle formula proof Archives

The Proof of the Tangent Half-Angle Formula

May 3, 2012 GB High School Mathematics, High School Trigonometry, Problem Solving and Proofs

In this post, we prove the following trigonometric identity:

$\displaystyle \tan \frac{\theta}{2} = \frac{\sin\theta}{1 + \cos \theta} = \frac{1 - \cos \theta}{\sin \theta}$ .

Proof

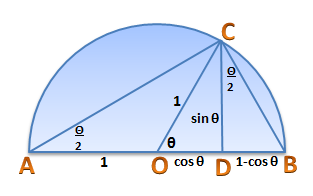
Consider a semi-circle with “center” $O$ and diameter $AB$ and radius equal to 1 unit as shown below. If we let $\angle BOC =\theta$ , then by the Inscribed Angle Theorem, $\angle CAB = \frac{\theta}{2}$ .

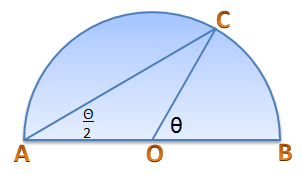
Draw $CD$ perpendicular to $OB$ as shown in the second figure. We can compute for the sine and cosine of $\theta$ which equal to the lengths of $CD$ and $OD$ , respectively. In effect, $BD = 1 - \cos \theta$ and $AD = 1 + \cos \theta$ . » Read more

2 comments half angle formula, half angle formula identity, half angle formula proof, inscribed angle theorem, proof without words, trigonometric identities, trigonometric identity