complex numbers Archives - Math and Multimedia

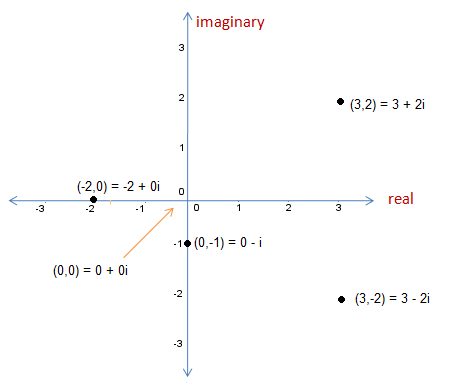
Introduction to the Complex Plane

February 12, 2012 GB High School Mathematics

Imaginary numbers had no use when it was invented except for intellectual exploration until it was linked to coordinate geometry. The complex number $a + bi$ can be plotted as the ordered pair $(a,b)$ where $a$ is the real part and $b$ is the imaginary part. Therefore, the complex numbers $3 + 2i$ , $3- 2i$ , $-2 + 0i$ , and $0 - i$ can be plotted as $(3,2)$ , $(3,-2)$ , $(-2,0)$ , and $(0,-1)$ in a “coordinate plane” respectively as shown below. In the figure, we can see that the origin is $0 + 0i$ and that $3 - 2i$ , the complex conjugate of $3 + 2i$ , is its reflection along the x-axis (Can this be generalized?).

The plane where the complex numbers are plotted above is called the complex plane.

In the complex plane, we can observe that all numbers of the form $(a,0)$ are real numbers and these numbers are represented by the horizontal axis. The numbers of the form $(0,bi)$ are all imaginary numbers and it is represented by the vertical axis. Thus, we can call them the real axis and the imaginary axis respectively.

In addition, we can conclude that all real numbers are complex numbers since for all real numbers $a$ , $a = a + 0i$ . So, the set of real numbers is a subset of the set of complex numbers.

Leave a comment complex numbers, complex plane, imaginary numbers, real numbers

Demystifying Complex Conjugates

February 2, 2012 GB High School Mathematics

This is the third post in the Complex Numbers Primer. In the previous post in this series, we have learned that complex numbers can be added and multiplied just like binomials. We did not discuss subtraction since it follows from addition; that is, if $a + bi$ and $c + di$ are complex numbers, then

$a + bi - (c + di) = a - c + (b - d)i$ .

Since we have completed the three fundamental operations on complex numbers, the next logical question would be how do we divide complex numbers? For example, how do we find the quotient of the expression

$\displaystyle\frac{5 + 2i}{3 - i}$ ?

Before we answer that question, recall that in simplifying rational expressions with radicals in the denominator, we multiply the numerator and the denominator of the expression by the ‘conjugate’ of the denominator. For instance, to simplify

$\displaystyle\frac{5}{2 + \sqrt{3}}$ . » Read more

One comment complex conjugates, complex numbers, real numbers

Complex Numbers and their Properties

January 28, 2012 GB High School Mathematics

Imaginary numbers as we have discussed in Tuesday’s post are numbers of the form bi where $b$ is a real number and $i = \sqrt{-1}$ . The term imaginary as (opposed to real) was first used by Rene Descartes, the mathematician who invented Coordinate Geometry — the Cartesian plane in particular. Leonhard Euler was the one who introduced the symbol $i$ for $\sqrt{-1}$ .

Leonhard Euler

Gerolamo Cardano, a pioneer in probability, was the one who suggested the use of numbers of the form $a + bi$ where $a$ and $b$ are real numbers and $i = \sqrt{-1}$ . Numbers of this form were named complex numbers by Carl Frederich Gauss.The real part of $a + bi$ is $a$ and the imaginary part is bi. » Read more

One comment carl frederich gauss, complex numbers, imaginary numbers, leonhard euler