Area Tutorial 4 – Derivation of the Area of a Circle
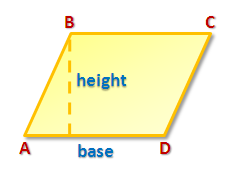
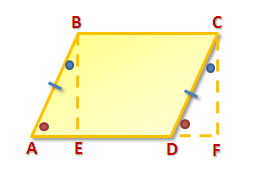
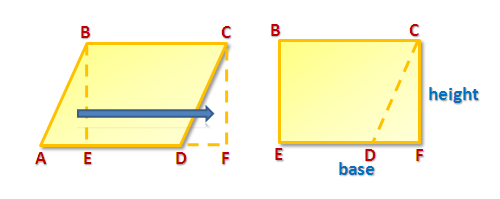
We have learned that the area of a parallelogram is the product of its base and its height, and the circumference of a circle with radius is
.
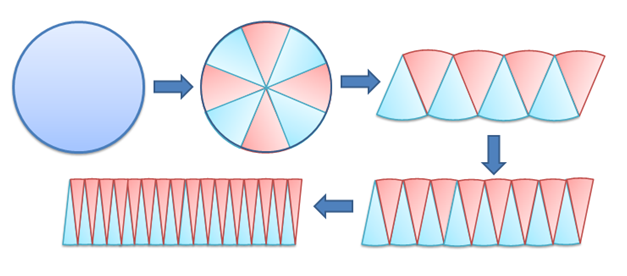
To find the area of a circle with radius , divide it into congruent sectors (blue and red divisions) then arrange them as shown below.
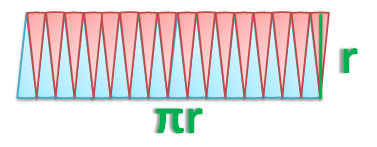
Observe that as the number of sectors increases, the shape of the rearranged sectors is becoming more and more like a parallelogram. In fact, if we can divide the circle into an infinite a number of sectors, it seems that the shape of the rearranged sector is a parallelogram. Assuming that this is true, then the base of a parallelogram is (Explain why.), and its altitude is
.
Since the area of a parallelogram is , we just have to multiply the base of the parallelogram which is
and its height which is
to find its area. Therefore, the area of the parallelogram, which is equal to the area of a circle, is
.
Another derivation
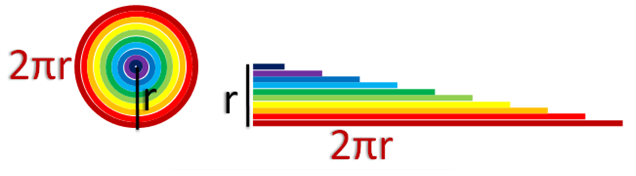
We can also derive the area of a circle by unwinding an infinite number of circular tracks. The smaller the width of our track becomes, the rearranged figure becomes more and more like a triangle. If it is indeed a triangle, then its area is the product of its height and its width.
Recall that the area of a triangle is the product of its base and height divided by 2. Since the base of the of the triangle is equal to the circumference of the circle (), and its height is equal to its radius (
), therefore, the area of the triangle, which is equal to the area of the circle, is
.
The processes that we have done above are logical; however, we only assumed that we can divide the circle into infinite number of sectors, or we can unwind an infinite number of tracks. These are just assumptions, hence, we are not yet sure if the area of the circle is indeed . Of course, we know it is true but we need a proof. The proof finding the area of a circle needs knowledge on integral calculus. We will discuss the proof of the area of the circle in the future.
Enjoy and Learn More