Month: March 2010

CaR Tutorial 2 – The MidSegment Theorem

Compass and Ruler, High School Geometry, Software Tutorials

In the previous CaR tutorial, we constructed and isosceles triangle. In this tutorial we are going to explore the properties of the segment connecting the midpoints of its two sides. In this tutorial we are going to learn the following:

  • use the move tool, triangle tool and segment tool
  • find the midpoint of two a segment
  • measure angles using the angle tool
  • edit properties and reveal measures of angles and segments

Construction Steps

1.) Open CaR. We will not need the Coordinate axes so click the Show grid icon until the Show the Grid icon until the grid or axes is not shown.
2.) Click the Triangle tool and click three different points on the drawing pad.
3.) Click the Move tool and right click one of the points to display the Edit Point dialog box. In the Name text box, change the name to A, then click the Show object names button (enclosed with red ellipse in Figure 1).

Figure 1 – The Edit Point dialog box.
4.) Change the name of the other two points to B and C.
5.) Click the midpoint tool, click point A and click point B to get the midpoint of AB. Now, get the midpoint of BC. Rename the midpoint of AB to E and the midpoint of AC to F (Refer to step 3). Your drawing should look like Figure 2.

Figure 2 – Triangle ABC with midpoints D and E.
6.) Right click and drag the labels to adjust their positions. Using the Move tool, move the vertices of the triangle. What do you observe?
7.) We will see the relationship of the angles and the segments in triangle ABC. We will measure the angle first. To measure angle ADE, click the points in the following order: point A, point B and point C. After this step, you will see the angle symbol at angle ADE.
8.) To display the measure of the angle, click the Move tool and right click the angle symbol. This will display the Edit Angle dialog box shown in Figure 2.
9.) To display the measure of the angle, click the Show object values icon. Then click the smallest angle symbol size to reduce the angle size. Now, click the OK button to apply changes.

Figure 3 – The Edit Angle dialog box.
10.)  Using step 8-9, measure angles ABC, ACB and AED. After measuring, your drawing should look like the figure below.
11.)  Using the Move tool, drag the vertices of the triangle. What do you observe?
12.)  Based on the measures of the angles shown in your drawing, what can you say about segment DE and segment BC?
13. ) Now, we will see if there is a relationship between the length of the segments in triangle ABC. To reveal the measure of DE, use the Move tool and right click the segment. This will reveal the Edit Line, Ray, Segment dialog box as shown in Figure 3.

Figure 5 - The Edit Line, Ray, Segment dialog box.
14.)  In the Edit Line, Ray, Segment dialog box, click the Show object values button.
15.)  Using steps 13-14, display the length of segment BC.
16.)  What can you observe about the relationship of segments DE and BC?
17.)  Move the vertices of the triangle. Are your observations still the same?
18.)  Make a conjecture about your observations above.
One comment , , , , , ,

Area Tutorial 2: Area of a Triangle

Elementary School Geometry, Elementary School Mathematics

In the previous area tutorial, we have learned that the area of a rectangle is equal to the product of its length and its width. For instance, the rectangle in Figure 1 has width 10 units and length 12 units, so its area is 120 square units. We have also learned in elementary school mathematics that the area of a triangle is half the product of its base length and its altitude.

Why is this so?

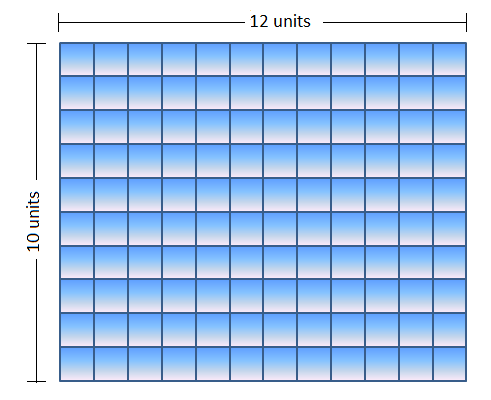
Figure 1 – Rectangle with length 12 units and width 10 units.

If we are going to relate the area of the triangle to the area of a rectangle  given its length and width, then the easiest to compute is the area of a right triangle. In Figure 2, the rectangle is divided into two congruent triangles, which implies that the area of the triangle is half of the area of the rectangle. Also, the rectangle’s length became the triangle’s base and the rectangle’s width became the triangle’s altitude. Hence, it is clear that the area of the right triangle below is half the product of the length of its base and its altitude.

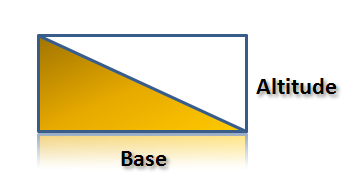
Figure 2 – The area of the right triangle is half the product of the base and the altitude of the rectangle.

The next question, however, is what if the triangle is not right? Does the formula still apply?

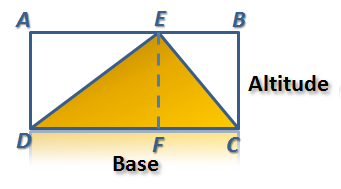
Figure 3 – The area of the non-right triangle above is still half the product of its base and its altitude.

In Figure 3, we have not changed the base and the altitude of the rectangle. If we draw a segment from the base to its opposite vertex (segment EF), then we form two smaller rectangles – rectangle AEFD and rectangle EFCB. From Figure 3, it is clear that the area of triangle EFD is half the area of rectangle AEFD. Also, the area of triangle EFC is half the area of rectangle EFCB. Thus, the area of triangle CDE is half the area of rectangle ABCD.

From the discussion above, we can conclude that if we can enclose a triangle with a rectangle with a given length (base) and width (altitude), then the area of that triangle is half the area of the enclosing rectangle.  This is with the condition that the base of the triangle coincides with one of the sides of the rectangle, and the vertex opposite the base touches the side of the rectangle that is parallel to the base.

Does the formula work for all triangles?

The two cases above involve acute and right triangles, so what we have left is an obtuse triangle as shown below.  As we can see, the vertex opposite the base is not touching the side of the rectangle that is parallel to the base.

One strategy in enclosing a triangle with a rectangle is to draw an altitude such that the altitude is inside the rectangle. In Figure 4, we cannot draw an altitude (perpendicular to the ground) inside the rectangle, so we will not be able to compute its area.

Figure 4 – An example of an obtuse triangle that cannot be enclosed by a rectangle using a particular side.

The remedy is shown in Figure 5. We change the base and change the altitude. In this case, the area of the triangle is half of the enclosing rectangle. This is true, since the condition above states that the length and width of the rectangle are given.

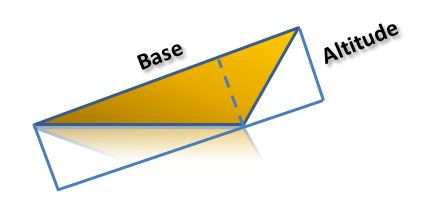
Figure 5 - Getting the area of a triangle by changing the base.

Delving Deeper

Playfair’s axiom guarantees that we can enclose any triangle with a rectangle, because given a line (base of a triangle) and a point (opposite vertex), we can always draw a unique line parallel to the base and passing through that vertex. To construct an enclosing rectangle, we can also draw two lines perpendicular to the base and passing through the other two vertices.

Enjoy and Learn More

6 comments , , , ,
1 … 3 4 5