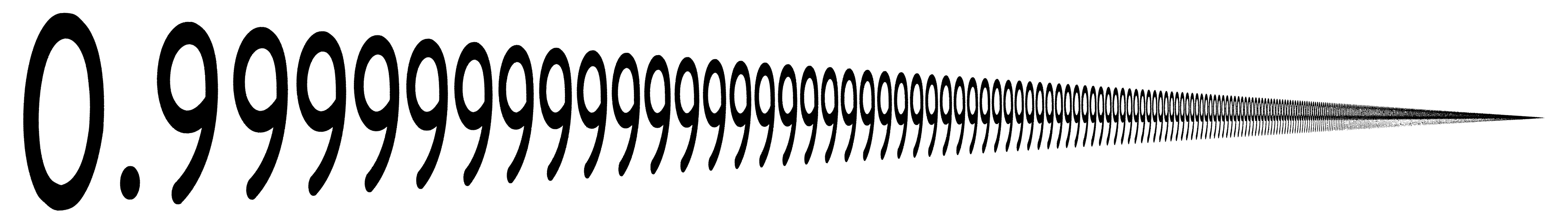Introduction
Yes it is. 0.999… is equal to 1.
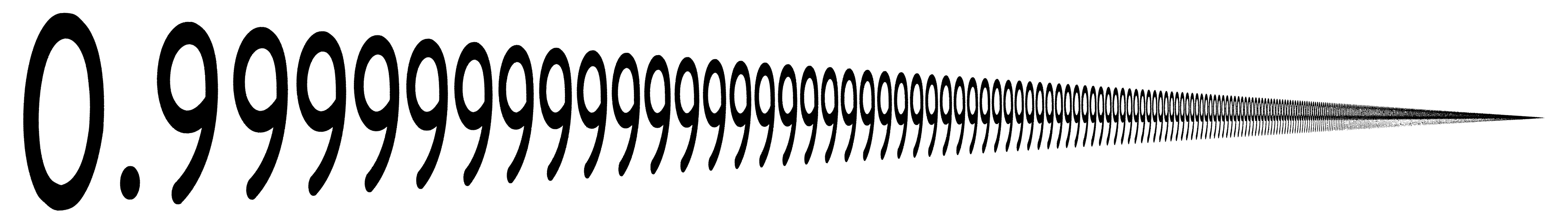
Before we begin our discussion, let me make a remark that the symbol “…” in the decimal 0.999… means that the there are infinitely many 9’s, or putting it in plain language, the decimal number has no end.
For non-math persons, you will probably disagree with the equality, but there are many elementary proofs that could show it, some of which, I have shown below. A proof is a series of valid, logical and relevant arguments (see Introduction to Mathematical Proofs for details), that shows the truth or falsity of a statement.
Proof 1

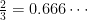
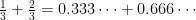

But 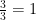 , therefore
, therefore 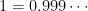
Proof 2

Multiplying both sides by 9 we have
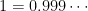
Proof 3
Let 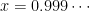

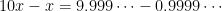

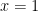
Hence, 
Still in doubt?
Many will probably be reluctant in accepting the equality 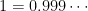 because the representation is a bit counterintuitive. The said equality requires the notion of the real number system, a good grasp of the concept of limits, and knowledge on infinitesimals or calculus in general. If, for instance,you have already taken sequences (in calculus), you may think of the
because the representation is a bit counterintuitive. The said equality requires the notion of the real number system, a good grasp of the concept of limits, and knowledge on infinitesimals or calculus in general. If, for instance,you have already taken sequences (in calculus), you may think of the  as a sequence of real numbers
as a sequence of real numbers  . Note that the sequence gets closer and closer to 1, and therefore, its limit is 1.
. Note that the sequence gets closer and closer to 1, and therefore, its limit is 1.
Infinite Geometric Sequence
My final attempt to convince you that  is indeed equal to
is indeed equal to 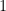 is by the infinite geometric sequence. For the sake of brevity, in the remaining part of this article, we will simply use the term “infinite sequence” to refer to an infinite geometric sequence. We will use the concept of the sum of an infinite sequence, which is known as an infinite series, to show that
is by the infinite geometric sequence. For the sake of brevity, in the remaining part of this article, we will simply use the term “infinite sequence” to refer to an infinite geometric sequence. We will use the concept of the sum of an infinite sequence, which is known as an infinite series, to show that  .
.
One example of an infinite series is  . If you add its infinite number of terms, the answer is equal to 1. Again, this is counterintuitive.
. If you add its infinite number of terms, the answer is equal to 1. Again, this is counterintuitive.
How can addition of numbers with infinite number of terms have an exact (or a finite) answer?
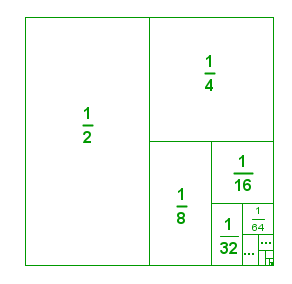
There is a formula to get the sum of an infinite geometric sequence, but before we discuss the formula, let me give the geometric interpretation of the sum above. The sum  can be represented geometrically using a 1 unit by 1 unit square as shown below. If we divide the square into two, then we will have two rectangles, each of which has area
can be represented geometrically using a 1 unit by 1 unit square as shown below. If we divide the square into two, then we will have two rectangles, each of which has area  square units. Dividing the other half into two, then we have three rectangles with areas
square units. Dividing the other half into two, then we have three rectangles with areas  ,
,  ,
,  square units. Dividing the one of the smaller rectangle into two, then we have four rectangles with areas
square units. Dividing the one of the smaller rectangle into two, then we have four rectangles with areas  ,
,  ,
,  ,
,  . Again, dividing one of the smallest rectangle into two, we have five rectangles with areas
. Again, dividing one of the smallest rectangle into two, we have five rectangles with areas  ,
,  ,
,  ,
,  , and
, and  Since this process can go on forever, the sum of all the areas of all the rectangles will equal to 1, which is the area of the original square.
Since this process can go on forever, the sum of all the areas of all the rectangles will equal to 1, which is the area of the original square.
Now that we have seen that an infinite series can have a finite sum, we will now show that  can be expressed as a finite sum by expressing it as an infinite series. The number
can be expressed as a finite sum by expressing it as an infinite series. The number  can be expressed as an infinite series
can be expressed as an infinite series 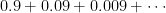 . Converting it in fractional form, we have
. Converting it in fractional form, we have  .
.
We have learned that the sum of the infinite series with first term 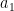 and ratio
and ratio  is described by
is described by  . Applying the formula to our series above, we have
. Applying the formula to our series above, we have

Therefore, the sum our infinite series is 1.
Implication
This implication of the equality  means that any rational number that is a non-repeating decimal can be expressed as a repeating decimal. Since
means that any rational number that is a non-repeating decimal can be expressed as a repeating decimal. Since 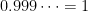 , it follows that
, it follows that 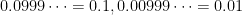 and so on. Hence, any decimal number maybe expressed as number + 0.00…01. For example, the decimal
and so on. Hence, any decimal number maybe expressed as number + 0.00…01. For example, the decimal 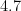 , can be expressed as
, can be expressed as  . The number
. The number  can also be expressed as
can also be expressed as 
Conclusion
Any of the four proofs above is actually sufficient to show that  . Although this concept is quite hard to accept, we should remember that in mathematics, as long as the steps of operations or reasoning performed are valid and logical, the conclusion will be unquestionably valid.
. Although this concept is quite hard to accept, we should remember that in mathematics, as long as the steps of operations or reasoning performed are valid and logical, the conclusion will be unquestionably valid.
There are many counterintuitive concepts in mathematics and the equality  is only one of the many. In my post, Counting the Uncountable: A Glimpse at the Infinite, we have also encountered one: that the number of integers (negative, 0, positive) is equal to the number of counting numbers (positive integers) and we have shown it by one-to-one pairing. We have also shown that the number of counting numbers is the same as the number of rational numbers. Thus, we have shown that a subset can have the same element as the “supposed” bigger set. I guess that is what makes mathematics unique; intuitively, some concepts do not make sense, but by valid and logical reasoning, they perfectly do.
is only one of the many. In my post, Counting the Uncountable: A Glimpse at the Infinite, we have also encountered one: that the number of integers (negative, 0, positive) is equal to the number of counting numbers (positive integers) and we have shown it by one-to-one pairing. We have also shown that the number of counting numbers is the same as the number of rational numbers. Thus, we have shown that a subset can have the same element as the “supposed” bigger set. I guess that is what makes mathematics unique; intuitively, some concepts do not make sense, but by valid and logical reasoning, they perfectly do.
Notes:
- You can find discussions about 0.999… = 1 here and here.
- There is another good post about it here and here.
Related Articles